linerprimo.blogg.se - Crc checksum

For example here a 6-bit pattern is replaced by 3 terms.

The benefits of using polynomial codes is that it produces short codes.

Figure shows the polynomial where all the terms with zero coefficient are removed and x J is replaced by x and XO by 1.

For example, if binary pattern is 100101, its corresponding polynomial representation is x 5 + x 2 + 1.

Here, the power of each term shows the position of the bit and the coefficient shows the values of the bit.

A pattern of Os and 1s can be represented as a polynomial with coefficient of o and 1.

(b) Appending the CRC to the end of the data unit should result in the bit sequence which is exactly divisible by the divisor. (a) CRC should have exactly one bit less than divisor.

The probability of error detection and the types of detectable errors depends on the choice of divisor.

CRC can detect all the burst errors that affect an odd number of bits.

The remainder obtained is 000 it means there is no error. This data is again divided by a divisor 1011.ĥ. At the receiver side, data received is 1001110.Ĥ. Thus in this case divisor 1011 is replaced by 0000.ģ. During this process of division, whenever the leftmost bit of dividend or remainder is 0, we use a string of Os of same length as divisor. String of 3 zeroes is appended to 1011 as divisor is of 4 bits.

For example, if data to be transmitted is 1001 and predetermined divisor is 1011.

If remainder is non-zero then there is an error in data and receiver rejects it. If the remainder of division is zero, receiver assumes that there is no error in data and it accepts it.ħ. The receiver on receiving it divides data unit + CRC by the same divisor & checks the remainder.Ħ. The data unit + CRC is then transmitted to receiver.ĥ. Now, string of n Os appended to data unit is replaced by the CRC remainder (which is also of n bit).Ĥ. original data + string of n as are divided by the divisor using binary division and remainder is obtained. The length of predetermined divisor is n+ 1.Ģ.

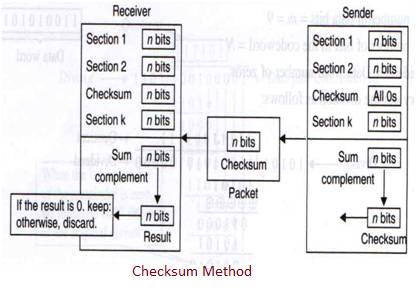
A string of n as is appended to the data unit. The various steps followed in the CRC method areġ.Appending the CRC to the end of the data unit should result in the bit sequence which is exactly divisible by the divisor. It should have exactly one less bit than divisor.Ģ. A sequence of redundant bits called CRC or CRC remainder is appended at the end of a data unit such as byte.Ī CRC will be valid if and only if it satisfies the following requirements:ġ. This technique is more powerful than the parity check and checksum error detection.If remainder after division is not zero, it indicates that the data unit has been damaged in transit and therefore it is rejected.If the remainder after division is zero then there is no error in the data unit & receiver accepts it.data + CRC is divided by the same number (predetermined binary divisor). At the destination, the incoming data unit i.e.The received frame is divided by P.īecause of no remainder, there are no errors. Suppose that there are no errors, and the receiver gets T perfect. The remainder is inserted to 2 5D to provide T = 101000110101110 that is sent. The message is generated through 2 5:accommodating 1010001101000 The CRC of n bits interpreted in phase 2 restores the added 0s at the end of the data unit. The new data unit is divided by a divisor utilizing a procedure known as binary division the remainder appearing from the division is CRC. The number n is one smaller than the number of bits in the fixed divisor. Joining it to the end of the data unit should create the resulting bit sequence precisely divisible by the divisor.Ī string of n 0s is added to the data unit.

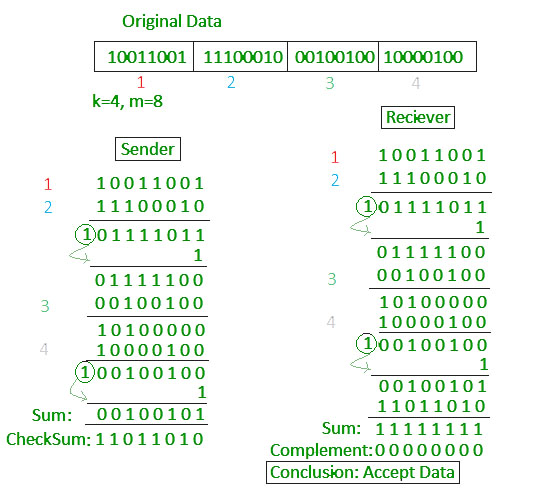
It should have accurately one less bit than the divisor. The redundancy bits used by CRC are changed by splitting the data unit by a fixed divisor. Modulo 2 Arithmetic is used in this binary addition with no carries, just like the XOR operation. The out coming frame, including n bits, is precisely divisible by some fixed number. It is given as a kbit message and the transmitter creates an (n – k) bit sequence called frame check sequence. The Cyclic Redundancy Checks (CRC) is the most powerful method for Error-Detection and Correction.